
There is general agreement that different capacitors can be demonstrated to have different sound qualities in audio applications under various conditions. Which capacitors in what circuits and under what conditions remains hotly debated. Not helping matters any, there is a tremendous amount of myth, lore and legend, plus marketing hype and defective test methodology out there that confuses the issue and causes a great divide between the objective and subjective camps of audio design philosophy.
Make no mistake, I sit solidly around the campfire with the objectivists. I have no tolerance for the viewpoint that sound quality is beyond our apparently primitive and useless measurement capabilities, that there is some "parameter X" we have yet to discover and that can't be measured with current technology. The cases that support such an argument invariably fall apart under a careful examination- measurements were never actually made, the wrong measurements were made or they were made poorly. Many times the claimed difference disappears under more strict listening conditions. It seems incomprehensible to the subjective camp that they're subject to all the same biases as the rest of us, but they are. I would never say that ferreting out sound quality differences is easy, in fact it can be notoriously difficult, but the tools and techniques do exist. The only item frequently in short supply is dogged perseverance.
I'm going to present test data for a small group of 0.01 uF capacitors to show first, that when people talk about "measurements" they're usually ignoring things that could affect sound quality and second, there are obvious measurable differences between all of the parts. Though I can't say at what level these differences become audible, I would strongly suggest that if two parts are very similar when subjected to a complete suite of tests, it can be said with almost complete certainty that, given a properly conducted listening test, their sound will be indistinguishable- regardless of cost, construction and flowery marketing prose. Unfortunately, it will also become apparent that every part type has its own distinguishing characteristics and the happy situation of equivalent parts arises less frequently than one would hope.
The capacitor group consists mostly of 0.01 uF film types because of the availability of a 0.01 uF Teflon capacitor I wanted to include in the tests (thanks Stefano!). A silver-mica and a ceramic are also included so that the line-up contains an example of most of the possible choices one might consider for a crossover or RIAA network. A few types were slightly different in value but close enough to compare for our purposes. Thus we have:

The test suite consisted of the usual low level capacitance and dissipation factor measurements at 1 kHz, series model. A simple
test for dielectric absorption was made, plus a few high frequency measurements. These would be the typical measurements one might
find on a data sheet. In addition, the stray capacitance to a metal plate was measured. Some "boutique" capacitors tend to be very
large and stray capacitance to surrounding objects like ground planes and chassis can become a concern, though the Audience cap
tested here is only slightly larger than a polystyrene. Finally, a new test for non-linearity and distortion was added to the
mix- nothing more than examining the bridge residual at null. More on that later. Note that I can easily think of half a dozen
or more measurements that I haven't made, so this should not be considered as any final word on capacitor measurement, only an
illustration that there's a lot more one can do besides the usual value and DF measurements.
| Brand | Audience | MIAL | Philips | Panasonic | Acushnet | Comp. Research | CDC | CGW | ASC x463 |
|---|---|---|---|---|---|---|---|---|---|
| Dielectric | Teflon | Polystyrene | Polystyrene | Polyprop | Polyester | Polycarb? | Silver-mica | Ceramic | Polycarb |
| Temperature | 72 | 72 | 72 | 72 | 72 | 70 | 72 | 73 | 72 |
| Voltage rating | 600 | 160 | 63 | 50 | 600 | 50 | 500 | 50 | 50 |
| Value, mrkd | 0.01 | 0.012 | 0.0095 | 0.0082 | 0.01 | 0.01 | 0.01 | 0.01 | 0.01 |
| Value, meas | 0.01006822 | 0.0122617 | 0.00952693 | 0.0082597 | 0.00929425 | 0.00988958 | 0.01000063 | 0.00999143 | 0.00982809 |
| Tolerance % | 1 | 2.5 | 1 | 2 | 10 | 1 | 1 | 10 | 10 |
| Error % | 0.68% | 2.18% | 0.28% | 0.73% | -7.06% | -1.10% | 0.01% | -0.09% | -1.72% |
| Dissipation | 0.000023 | 0.000053 | 0.000028 | 0.000122 | 0.004739 | 0.000791 | 0.000168 | 0.000108 | 0.000705 |
| Absorption | 0.02% | 0.02% | 0.02% | 0.04% | 0.23% | 0.02% | 0.82% | 0.34% | n/a |
| DCR, 100V | 6.50E+13 | 3.00E+13 | 2.00E+15 | 3.50E+14 | 9.50E+10 | 1.50E+14 | 2.00E+12 | 3.00E+12 | n/a |
| Phase, 2MHz | -84 | -84 | -84 | -86 | -84 | -83 | -86 | -84 | n/a |
| R, 2MHz | 6.35 | 6 | 7.8 | 9.2 | 8.5 | 7.8 | 7.6 | 7.6 | n/a |
| Self-res, MHz | 4.5 | 7 | 7.7 | 9.7 | 7.5 | 9.7 | 8.4 | 9.2 | n/a |
| Stray C | 5.0 | 2.6 | 4.4 | 3.8 | 4.6 | 2.8 | 6.0 | n/a | n/a |
| Bridge residual | low | low | low | very low | high | high | low | low | high |
In a perfect world, you'd expect a capacitor to match its marked value exactly, with most other parameters being zero or infinity. The basic capacitance measurements here are unremarkable, as the parts mostly meet tolerance. All film capacitors have a significant temperature coefficient, so it's a bit silly to measure them on a standards bridge at part per million levels except for the fact that this same bridge allows very accurate dissipation measurements on nearly perfect dielectrics like Teflon. Much time was spent waiting for the capacitance value to stabilize after so much as touching the leads, which raises the temperature. One should consider the temperature coefficient when constructing an RIAA network and calculate the deviation when the capacitors go from turn-on on a cold day, to fully warmed up on a hot summer day. Most preamps don't have ventilation, and this would be a reason to consider it.
Dissipation factor is useful when evaluating the health of power supply electrolytics. It's effect on the sonic performance of signal capacitors isn't agreed on, but may be quite minor. The number represents internal losses, and can be converted to effective series resistance (ESR) if desired. ESR is not constant with frequency, but tends to be so low in high quality capacitors that it doesn't have much effect on circuit performance. If one were building high Q resonant circuits it would be a different story. Still, low dissipation factor seems to be a hallmark of good dielectrics, so a high number can be a tip off that more investigation is needed.
Dielectric absorption is possibly more disturbing. There is remarkably little published on the matter. It was a significant issue with early analog computers and some related books covered it. There was also a good paper by Bob Pease, published by National Semiconductor, that may be the best write up to date. I'll speculate that high dielectric absorption is not always serious, since silver-mica capacitors suffer from an excessive amount of it, yet can produce very good sounding RIAA networks. In later measurements of residual distortion, high dielectric absorption doesn't produce high residuals, but I'll still remain suspicious of it until it's proven harmless.
DC leakage measurements should have no effect on anything, as resistance should be very high in any signal capacitor. They do indicate one interesting thing about capacitor construction. With higher dielectric constant materials, less surface area is required and leakage can be almost unmeasurably low. Lower dielectric constant materials like Teflon, in spite of its basic high resistivity, may require so much surface area that leakage, possibly from the slightest contamination or impurity, can increase. DC leakage is probably a good quality control measurement, but not relevant to sound. Note that lower voltage rated caps were measured at 50 VDC instead of 100 VDC.
Film capacitors generally have excellent high frequency capabilities but this is often compromised by large size and long lead lengths. You'll notice that the small radial Panasonic capacitor has a much higher self resonance (9.7 MHz) than the Audience capacitor (4.5 MHz). This isn't due to any failing of the high end Teflon cap, merely the fact that it has several inches of lead length and couldn't be connected near the body. The Panasonic cap has a fraction of an inch of lead length, and the body is smaller. If you need high frequency performance, possibly not for sound, but to maintain stability with high bandwidth semiconductors, keep size and lead length to an absolute minimum. For bypass duty, surface mount will beat leaded every time.
An often neglected parameter is how much capacitance a device presents to nearby objects. A physically large capacitor will have a significant capacitance between it's outer electrode and surrounding parts. There may be undesired coupling to a ground plane. Less appreciated is that the capacitor may not be surrounded with the same wonderful dielectric it was built with. It may well be wrapped with polyester, regardless of what the dielectric is. Thus, the quality of the unwanted capacitance may be poor. It would take a better setup (strict shielded 3-terminal connections to an enclosed measurement chamber) to get a reliable DF number for these low values, so that was ignored on this go around.
Inexperienced designers often simulate circuits in Spice, but rarely do they include the extra capacitive and inductive couplings that exist in real physical circuits. There's no substitute for bench experience. If you're building a precision network be sure to account for strays. The capacitance between a metal plate and the capacitor under test was measured with the cap leads shorted and the largest face of the capacitor in contact with the metal plate. The Philips part was a special case as the pair couldn't be placed in good contact with the plate. A separate Philips cap was tried on all surfaces for the maximum stray value, then the value doubled to represent the pair. All measurements were 1 kHz, series model. All the caps tested showed only a few pF to the plate, so in any normal audio frequency network application there would be little to worry about. If a cap with similar stray body capacitance were used across an opamp feedback resistor, or on the very high impedance grid of a tube, this amount of capacitance would need to be carefully considered. (yes, I forgot to measure the ceramic but as the body is small, the capacitance should also be small.)
The last item to talk about is bridge residual. This was measured using a Schering Bridge, an old General Radio Corp. 716C. This was chosen because the major adjustable impedances in the bridge arms are both high quality air capacitors. There are also some range and trim parts that use polystyrene and silver-mica capacitors, but overall the bridge contributes almost no non-linearity or distortion to a measurement.
Normally a traditional bridge is balanced using a tuned null detector. A single frequency is fed in and all but that frequency is filtered out when looking at the output. In that way the signal to noise ratio is tremendous and measurements can be made to high precision without worrying about hum and noise.
The bridge still works just fine without filtering the output, in fact early bridges were balanced using nothing more than sensitive headphones as a detector. Without filtering, one notices a curious phenomenon. Some capacitors can be balanced to a much deeper null than others. It means that the capacitor doesn't perfectly adhere to the standard model for a capacitor, a perfect reactance in series or parallel with a perfect resistive loss element, but distorts the signal slightly.
This may be another manifestation of dielectric absorption specific to the measurement frequency (or it may not), but it turns out to be a very useful way of looking at capacitor distortion. The usual tests for dielectric absorption are cumbersome and hard to carry out at the frequencies most interesting to audio folk, but this is not. As you look at the following scope signals you'll notice that they don't correlate well with the very low frequency dielectric absorption measurements already made (or much of anything else), but I believe they may have some relation to perceived sound, at least in the case of polyester (Mylar). I believe Bates did several articles on capacitor distortion in the UK publication Electronics and Wireless World and it would be interesting to see if the results bear any similarity to these.
New Info! Does this tell us anything new? Probably not. After some discussion on one of the audio forums a member did more tests using a similar setup. It appears an unaccounted for variable is the distortion of the signal generator supplying the bridge. I've yet to do my own test, but if you remove that variable and use a very clean generator, the residual will track dissipation factor. Thus, you can probably ignore this residual stuff unless you find it interesting.
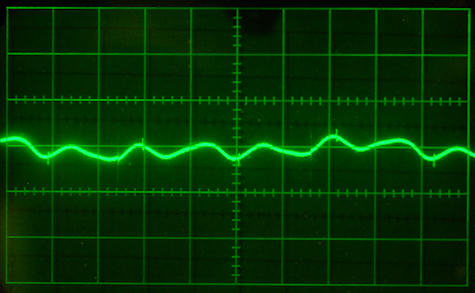
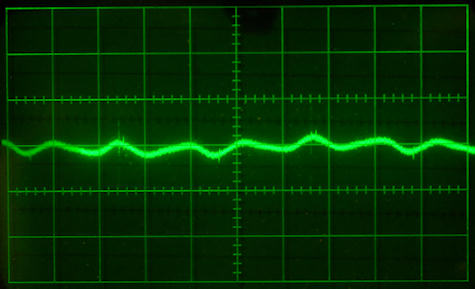
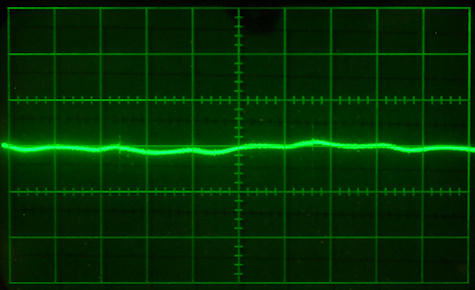
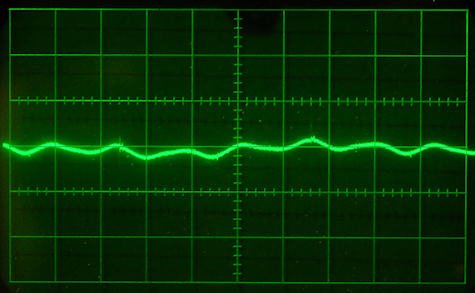
The measurement setup was identical for all caps. The bridge was excited with 120 Hz at about 40 VRMS which gives about 3.6 VRMS across the capacitor under test. The output of the bridge was viewed with a high gain scope plug-in set to 0.5 mV/division. Thus, all the scope images can be compared directly. The residual for all caps tested was 360 Hz, the 3rd harmonic. This is obviously "the nature of the beast", but is a bit counter intuitive and rather undesirable. Various other caps were tested and the results were consistent by type, i.e. by dielectric material.
Here is the bridge residual for the Audience Teflon cap:
Next the Philips polystyrene:
Next the Panasonic polypropylene:
Next the CDC silver-mica:
And finally, the Acushnet polyester (Mylar):
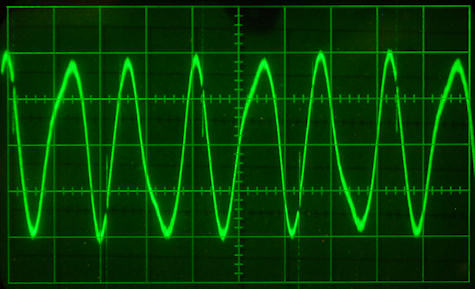
If nothing else, this may explain the consistent dislike for polyester capacitors in the high end audio community. As for the rest, I have to wonder if there's a preference for a non-zero amount of 3rd harmonic distortion, and that possibly "cap-rolling" is an attempt to hit a specific preference. Is it possible that at one end (polyester) we get the phrase, "hard and gritty", and at the other end (polypropylene) we get, "sucks the life out of the music"? Perhaps somewhere in the middle (Teflon?) lies just the right amount of "edge"? The engineer in me wants to reject all of this because the residuals are so small, but when you have a huge amount of anecdotal evidence, it's wise to at least speculate. I believe this would also be consistent with the almost universal high end rejection of amplifiers having near zero harmonic output, though admittedly the ability of people to distinguish different amplifiers in double blind testing has been shown nearly impossible.
Conrad R. Hoffman
Rev. 1, 6/6/2010
Edit 4/30/2022
6/7/2010 I'm not completely comfortable with ranking the caps based on the bridge residual. Unlike amplifier THD measurements, where it's almost impossible for distortions to cancel, a bridge is based on cancellation between two "identical" paths and might allow cancellation of internal and external errors. Thus, I have to wonder if there is some slight contribution by the bridge that can change the ranking between the nearly perfect caps. It just eats at me that the Teflon isn't the best of the bunch. There's no chance of that concerning the polyester cap, which is just plain bad, but I need to find a way to confirm "zero". Ideally that would be a giant 0.01 uF air capacitor, but such a thing doesn't exist. 0.001 uF is the largest air cap I have, and I want to confirm the readings on the same scale as the original measurements. It may be possible to perform the same measurement with the GR1615A standards bridge, which uses only air reference caps and a ratio transformer, though keeping hum and noise pickup down is a bit tougher with that unit.
6/14/2010 Hooking up 3 big GR air capacitors in parallel gave about 0.0035 uF, allowing a test to be made on the same range as the test group. Hum contamination was a huge issue since no shielded cables could be used- the cables would be an additional dielectric that would affect the results. The test was inconclusive as there was still a small residual, similar to that seen with the better caps in the test group. Another test was run using the 0.01 uF range extender capacitor from the standards bridge. This is well shielded and the residual was below anything visible at the scale the tests were run at. It is, however, not an air capacitor. It's probably polystyrene so the same question of canceled distortions remains. It appears that hum pickup can confuse things greatly so the frequency was changed to 200 and 400 Hz to separate the effects. More caps were checked with the following conclusions:
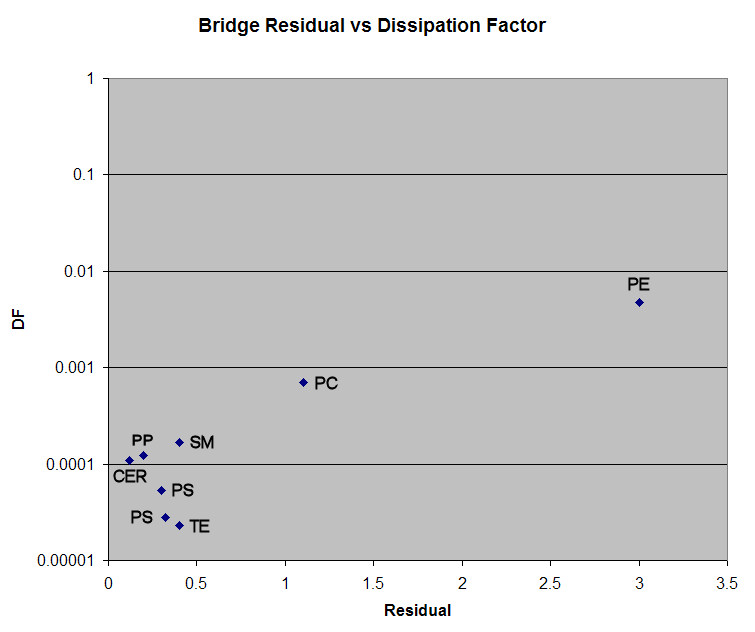
6/15/2010 Made a big effort to reduce hum pickup by disconnecting nearby equipment from the line and putting a complete shield over the test area of the bridge. I added an ASC x463 polycarbonate cap and a General Radio calibration cap (huge silver mica foil stack design- nothing to write home about for audio use). This test was at 60 Hz because the residual seems to be larger at low frequencies. It's very non-linear with test voltage- need to investigate that further. Here is a new ranking- the numbers are in mV of residual signal.
| ceramic | 0.12 |
| polypropylene | 0.2 |
| polystyrene (Philips) | 0.3 |
| polystyrene (MIAL) | 0.32 |
| Teflon | 0.4 |
| silver-mica | 0.4 |
| silver-mica (GR cal.) | 0.6 |
| x463 polycarbonate | 1.1 |
| polyester (Mylar) | 3.0 |
An Excel scatter plot showed no corelation with dielectric absorbtion, but some caps do fall on a line against dissipation factor. Note that DF is plotted on a log scale. The polystyrene and Teflon didn't fall on this line, as shown below.
7/11/15 Found CGW ceramic cap data and added to description. It's a unique cap of exceptionally low temperature coefficient and stability, sadly discontinued.
7/19/15 It was suggested by Henry P. Hall (ret. from GR, designed the Digibridge) that I check the caps for voltage coefficient and dielectric absorption. Setting up the 716-C with the GR recommended bias adapter, the change in capacitance was nearly unmeasurable for all the caps in question. The Mylar cap was about 0.0001%/volt using a 250 VDC bias. That doesn't seem like the source of any problem so at some point I'll do better DA tests.